Thermodynamics of Formation of Al₆Mn Inter-Metallic Compound for Mn Removal from Molten Al–Mg Alloy

Abstract

To reduce CO₂ emission, recycling Al scrap into wrought alloy is desired. However, impurity elements are inevitably contained in the Al scrap recovered from the society, and most of them are difficult to be removed by the current pyro-metallurgical processes. Mn is one of the major alloying elements of Al alloys and steels and is contained in Al scraps. Therefore, a removal method of Mn from Al is necessary. Mn easily forms an inter-metallic compound with Al; on the other hand, it is immiscible with Mg in the liquid state, which suggests that the repulsive interaction between Mg and Mn in molten Al enhances the precipitation of intermetallic compounds such as Al₆Mn. This study proposed Mn removal from molten Al–Mg alloy through precipitation of Al₆Mn inter-metallic compound. Molten Al–Mg alloy was equilibrated with Al₆Mn, and the reducing limit of Mn concentration was thermodynamically discussed. Mn concentration becomes lower at higher Mg content of molten Al and lower temperature. The activity coefficient of Mn in molten Al–Mg alloy was increased with the addition of Mg, and the following equation was obtained as a function of temperature and molar fraction of Mg:

It was found that Al₆Mn will precipitate due to the repulsive interaction of Mn and Mg when the Mg content of Al is increased. The thermodynamic analysis showed the possibility of reducing the Mn content of Al to 0.0030 mass% at 733 K and 34 mass%Mg by the present removal process.

Fig. 6 Contour lines of Mn content of molten Al–Mg alloy equilibrated with Al₆Mn.

1. Introduction

Recycling Al scrap is essential to achieve carbon neutrality because the amount of CO₂ emission can be decreased to 3% of the production process of Al from natural resources.^1–3) However, impurity elements are inevitably contained in the Al scraps recovered from society. Most of them are difficult to be removed by such current pyro-metallurgical processes as oxidation, gasification, and chlorination.^4–6) Because the tolerance level of impurity contents of wrought Al alloys is much lower than cast alloys, Al scrap is mainly recycled into cast Al alloys, which are used for engines and transmissions of automobiles.^2,7,8)

The electric car has been developed and will become more popular in the future. Therefore, the demand for automobile engines and transmissions will decrease, leading to a surplus of Al scraps.⁹⁾ Accordingly, recycling Al scrap into wrought alloys is expected.³⁾ Mn is one of the major alloying elements of Al alloys and steels. Therefore, Mn is often contained in the recovered Al scraps, and a removal method of Mn from Al is needed. Regarding the purification of Al, electro slag refining,¹⁰⁾ the segregation process,¹¹⁾ and the precipitation method^12–14) have been studied. The precipitation method is a molten alloy purification process through the precipitation of intermetallic compounds. However, Otaki et al.¹²⁾ reported that the precipitation did not occur when the concentration of impurity elements was low. Considering the repulsive interaction between Fe and Mg, Shinomiya et al.¹⁵⁾ proposed the removal method of Fe through the precipitation of Al₃Fe intermetallic compound by adding Mg in molten Al, which contains Fe. Sekii et al.¹⁶⁾ have also reported the removal method of Si in molten Al through the precipitation of Mg₂Si intermetallic compound by adding Mg. Mn easily forms an intermetallic compound with Al; on the other hand, it is immiscible with Mg in the liquid state, which suggests that the repulsive interaction between Mg and Mn in molten Al enhances the precipitation of intermetallic compounds. Mg is an essential element in 5000-series aluminum alloys, and Al–Mg alloys with a higher Mg content due to Mn removal may be used as an Mg source for producing such Al–Mg alloys. Mg in Al is also an element that can be removed by gasification, oxidation or chlorination,^4,6) if necessary. Accordingly, in this study, Mn removal from molten Al–Mg alloy through the intermetallic compound precipitation was proposed. According to the phase diagram of the Al–Mn binary system,¹⁷⁾ Al₆Mn coexists with the liquid phase at the melting point of Al. Therefore, molten Al–Mg alloy was equilibrated with Al₆Mn, and the solubility of Mn was investigated. From the experimental results, thermodynamic data of the formation of Al₆Mn inter-metallic compounds were derived. The reduction limit of Mn concentration was analyzed using the derived thermodynamic data.

2. Experimental Procedure

Al₂O₃ crucible (o.d.: 39 mm, i.d.: 35 mm, height: 37 mm) containing 30 g of Al (99.99%) and 10 g of Mn (99.9%) was put in a high-frequency induction furnace. The samples were heated to 1300 K in an Ar at a flow rate of 300 cm³/min(s.t.p.) and held for 10 min to be melted. Then, the heating was stopped, the sample was cooled in the furnace, and Al–Mn alloy was obtained.

The Al₂O₃ crucible containing Al and Mg (99.9%) was put in the high-frequency induction furnace. The samples were heated to 873 K in 50 vol%H₂–50 vol%Ar gas at a flow rate of 400 cm³/min(s.t.p.) and held for 10 min to be melted. Then, the heating was stopped, the sample was cooled in the furnace, and Al–Mg alloy was obtained.

The equilibrium experiments were conducted with an electric resistance furnace, shown in Fig. 1. A mullite tube sealed with silicone rubbers was vertically positioned at the center. Ar gas was introduced through an Al₂O₃ tube attached to the silicone rubber. The furnace temperature was measured with Pt–30Rh – Pt–6%Rh thermocouple and controlled with a P.I.D. controller. The experimental conditions are shown in Table 1. Al₂O₃ crucible (o.d.: 29 mm, i.d.: 24 mm, height: 51 mm) containing Al–Mn and Al–Mg alloys prepared preliminary was put in the mullite tube. The samples were heated to 823 or 873 K at 10 K/min and held for 19 h in Ar at a flow rate of 200 cm³/min(s.t.p.). Then, the crucible was withdrawn from the furnace and cooled in the air. The sample after the experiment was cut and taken for chemical analysis. Mg and Mn contents of the Al–Mg alloy phase were analyzed by an inductively coupled plasma–atomic emission spectrometry (ICP–AES). The compound phase of the sample was investigated by the X-ray diffraction method (XRD).

Fig. 1

Schematic of Experimental apparatus and arrangement of samples.

Table 1 Initial conditions of equilibrium experiments.

3. Results and Discussion

3.1 Equilibrium composition of manganese in molten aluminum

Experimental results are summarized in Table 2, and the relationship between Mn and Mg content of molten Al–Mg alloy is shown in Fig. 2, together with their initial concentrations. The compound phase of the samples (No. 1, 3 and 6) was investigated by XRD and was identified to be Al₆Mn. Mn content of the alloys increased from zero due to the dissolution of the Al–Mn alloy. The Mn concentration becomes lower at higher Mg content of the alloy and lower temperature, which indicates that the Mn content of molten Al can be decreased with increasing Mg content and lowering the temperature. The maximum value of the Mn concentration was 1.79 mass% at 873 K and 12.8 mass%Mg, and the minimum value was 0.07 mass% at 823 K and 38.2 mass%Mg.

Table 2 Experimental results.

Fig. 2

Relationship between Mn and Mg contents of molten Al alloy.

3.2 Thermodynamics of formation of Al₆Mn inter-metallic compound

The formation of the Al₆Mn intermetallic compound was thermodynamically discussed. The equilibrium of molten Al–Mg alloy and Al₆Mn(s) is expressed as eq. (1).   

\begin{align} &\text{6Al($l$, in Al–Mg alloy)} + \text{Mn($l$, in Al–Mg alloy)}\\ &\quad = \text{Al$_{6}$Mn(s)} \end{align} (1)

Chemical species in this system are Al, Mg, Mn, and Al₆Mn. Therefore, the number of independent valuables is 4 − 1 = 3. Because 2 phases, Al–Mg and Al₆Mn, exist, the degree of freedom is calculated as 3 − 2 = 1 under constant temperature and pressure. Therefore, only one variable can be arbitrarily determined. In the following discussion, the Mg content of Al–Mg alloy was considered to be the variable. The Gibbs energy change of the reaction (1), $\Delta G_{(1)}^{\text{o}}$, was derived from the Gibbs energy data of Al₆Mn(s), Al(l) and Mn(l).¹⁸⁾   

\begin{equation} \Delta G_{(1)}^{\text{o}} = -189344 + 116.33T\quad (\text{J/mol}) \end{equation} (2)

  

\begin{equation} \ln K_{(1)} = -\frac{\Delta G_{(1)}^{\text{o}}}{RT} = \ln \left(\frac{a_{\text{Al${_{6}}$Mn}}}{a_{\text{Al}}{}^{6}\cdot a_{\text{Mn}}} \right), \end{equation} (3)

where T is the temperature (K), R is the gas constant (J/mol·K), and a_Al and a_Mn denote activities of Al and Mn relative to each pure liquid. $a_{\text{Al}_{6}\text{Mn}}$ is activity of Al₆Mn relative to pure solid, which is unity in this work. Because the activity of Mn is expressed as the product of the Raoultian activity coefficient and molar fraction, eq. (3) is rearranged as eq. (4).   

\begin{equation} \ln \gamma_{\text{Mn}}^{\text{o}} = -6\ln a_{\text{Al}} - \ln x_{\text{Mn}} - \ln K_{(1)} \end{equation} (4)

The activity coefficient of Mn in molten Al–Mg alloy can be derived from the activity of Al and the molar fraction of Mn in the alloy. Because Mn was dilute in the experimental sample alloys, as shown in Table 2, the activity coefficient of Mn was expressed as $\gamma_{\text{Mn}}^{\text{o}}$, which indicates the value for the infinitely dilute solution. Assuming the effect of Mn on the activity of Al can be negligible, the activity of Al was estimated from the thermodynamic data in Al–Mg binary system.¹⁹⁾ When molten Al–Mg alloy is considered to be the regular solution, the activity coefficient of Al can be expressed as eq. (5)   

\begin{equation} \ln \gamma_{\text{Al(${l}$,in Al–Mg alloy)}} = \alpha x_{\text{Mg}}{}^{2} \end{equation} (5)

Accordingly, the activity of Al is calculated by eq. (6).   

\begin{equation} a_{\text{Al}} = (1 - x_{\text{Mg}})\cdot \exp (\alpha x_{\text{Mg}}{}^{2}) \end{equation} (6)

Furthermore, the relationship of eq. (7) can be applied to the regular solution because T ln γ_i = const. (i: Al or Mg). Using eq. (7), the value of the activity coefficient at a given temperature can be calculated from the value measured at temperature T₀.   

\begin{equation} \ln \gamma_{i} (T) = \frac{T_{0}}{T}\ln \gamma_{i} (T_{0}) \end{equation} (7)

Shinomiya et al.¹⁵⁾ estimated α = −0.459 at 873 K, and Sekii et al.¹⁶⁾ estimated α = −0.487 at 823 K from the thermodynamic data in Al–Mg binary system at 1000 K¹⁹⁾ using eq. (7). Equation (8) can be derived from eq. (4) and (6).   

\begin{equation} \ln \gamma_{\text{Mn}}^{\text{o}} = -6\{\alpha x_{\text{Mg}}{}^{2} + \ln (1 - x_{\text{Mg}})\} - \ln x_{\text{Mn}} - \ln K_{(1)} \end{equation} (8)

The values of the activity of Al and the activity coefficient of Mn are calculated by eqs. (6) and (8), respectively, and are shown in Table 3. Figure 3 shows the effect of the Mg content of molten Al–Mg alloy on the activity coefficient of Mn. The activity coefficient of Mn becomes larger at higher Mg content, which indicates that Mn and Mg repel each other in molten Al.

Table 3 Derived activity coefficient of Mn in molten Al–Mg alloy.

Fig. 3

Dependence of activity coefficient of Mn in molten Al–Mg alloy on Mg content at 823 and 873 K.

On the other hand, when Mn is diluted in Al–Mg–Mn ternary solution, the activity coefficient of Mn can be estimated by Toop’s equation expressed as eq. (9).^20–22)   

\begin{align} \ln \gamma_{\text{Mn(${l}$,in Al–Mg alloy)}}^{\text{o}} & = \frac{x_{\text{Al}}}{x_{\text{Mg}} + x_{\text{Al}}} \cdot \ln \gamma_{\text{Mn(${l}$,in Al)}}^{\text{o}} \\ & \quad + \frac{x_{\text{Mg}}}{x_{\text{Mg}} + x_{\text{Al}}}\cdot \ln \gamma_{\text{Mn(${l}$,in Mg)}}^{\text{o}}\\ & \quad -(1 - x_{\text{Mn}})^{2} \cdot \frac{\varDelta G_{\text{Al–Mg}}^{Ex}}{RT} \end{align} (9)

where $\gamma_{\text{Mn}(l,\text{in}\,\text{Mg})}^{\text{o}}$ and $\gamma_{\text{Mn}(l,\text{in}\,\text{Al})}^{\text{o}}$ denote the activity coefficient of Mn at infinitely dilute solution in molten Mg and Al, respectively. $\varDelta G_{\text{Al–Mg}}^{Ex}$ is an excess Gibbs energy of mixing in Al–Mg binary system. Because Mn is dilute, x_Mg + x_Al is approximated to be 1. Assuming the molten Al–Mg alloy is the regular solution, eq. (9) can be rearranged as eq. (10).¹⁵⁾   

\begin{align} \ln \gamma_{\text{Mn(${l}$,in Al–Mg alloy)}}^{\text{o}} &= \alpha x_{\text{Mg}}{}^{2} + (\ln \gamma_{\text{Mn(${l}$,in Mg)}}^{\text{o}} \\ & \quad - \ln \gamma_{\text{Mn(${l}$,in Al)}}^{\text{o}} - \alpha)x_{\text{Mg}}\\ & \quad + \ln \gamma_{\text{Mn(${l}$,in Al)}}^{\text{o}} \end{align} (10)

When parameters $A = \ln \gamma_{\text{Mn}(l,\text{in}\,\text{Mg})}^{\text{o}} - \ln \gamma_{\text{Mn}(l,\text{in}\,\text{Al})}^{\text{o}} - \alpha $ and $B = \ln \gamma_{\text{Mn}(l,\text{in}\,\text{Al})}^{\text{o}}$ are defined, eq. (10) is reduced to eq. (11).   

\begin{equation} \ln \gamma_{\text{Mn(${l}$,in Al–Mg alloy)}}^{\text{o}} - \alpha x_{\text{Mg}}{}^{2} = Ax_{\text{Mg}} + B \end{equation} (11)

Equation (11) indicates the linear relationship between the left-hand side and x_Mg. This relationship is shown in Fig. 4. The values of the activity coefficient of Mn at 823 K were converted to those at 873 K using eq. (7). A solid line is a linear approximation of all the plots, obtained by the least square method. From the slope and intercept of the approximation line, the values of the parameters, A = 17.94 and B = −8.80, were obtained, and $\ln \gamma_{\text{Mn}(l,\text{in}\,\text{Mg})}^{\text{o}} = 8.67$ and $\ln \gamma_{\text{Mn}(l,\text{in}\,\text{Al})}^{\text{o}} = - 8.80$ were derived. The thermodynamic data of the liquid phase in Mg–Mn²³⁾ and Al–Mn¹⁸⁾ binary systems have been derived by applying the Redlich-Kister type polynomial to calculate an excess Gibbs energy of mixing, which is expressed as eq. (12).²³⁾   

\begin{equation} \varDelta G_{i-\text{j}}^{Ex} = x_{i}x_{j}\sum_{\nu} L_{ij}^{\nu} (x_{i} - x_{j})^{\nu} \end{equation} (12)

where ν is an integer not less than 0 and $L_{ij}^{\nu }$ is an interaction parameter of ν-th order (J/mol). Satoh et al.²⁴⁾ have derived the relationship between the interaction parameters and the activity coefficient of an element at the infinitely dilute solution as eq. (13).²⁴⁾   

\begin{equation} \ln \gamma_{j}^{\text{o}} = \frac{L_{ij}^{0} + L_{ij}^{1}}{RT} \end{equation} (13)

Table 4 shows the interaction parameters in the liquid phase in Mg–Mn²³⁾ and Al–Mn¹⁸⁾ binary systems. Using eq. (13), the activity coefficients of Mn at 873 K were estimated to be $\ln \gamma_{\text{Mn}(l,\text{in}\,\text{Mg})}^{\text{o}} = 4.14$ and $\ln \gamma_{\text{Mn}(l,\text{in}\,\text{Al})}^{\text{o}} = - 6.23$ from the values in Table 4. The estimated activity coefficient of Mn in molten Mg is positive, whereas molten Al is negative, indicating that Mn and Mg repel whereas Mn and Al attract each other. This trend is consistent with the values derived from the experimental results. The thermodynamic discussion revealed that the solubility of Mn is decreased at higher Mg content because the activity coefficient of Mn increased due to the repulsive interaction of Mn and Mg when the Mg content of Al was increased.

Fig. 4

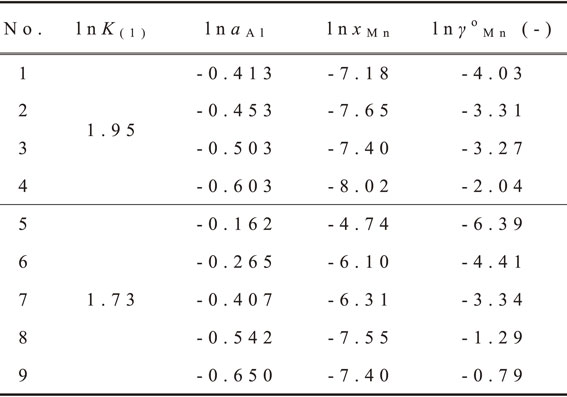
Determination of the activity coefficient of Mn in molten Al–Mg alloy at 873 K (the values of activity coefficient measured at 823 K were converted to those at 873 K using eq. (7)).

Table 4 Thermodynamic parameters in Mg–Mn²³⁾ and Al–Mn¹⁸⁾ binary systems.

From eqs. (5) and (10), the activity coefficient of Mn in molten Al–Mg alloy was derived as a function of temperature and molar fraction of Mg as the following equation:   

\begin{align} &\ln \gamma_{\text{Mn(${l}$,in Al–Mg alloy)}}^{\text{o}} \\ & \quad = \frac{873}{T}(-0.459x_{\text{Mg}}{}^{2} + 17.94x_{\text{Mg}} - 8.80) \end{align} (14)

The activity coefficient of Mn in molten Al–Mg alloys was calculated using eq. (14) and is shown in Fig. 3.

3.3 Thermodynamic analysis on Mn removal from molten Al–Mg alloy

Using the thermodynamic data derived in Section 3.2, the reducing limit of Mn concentration in this method was analyzed. Equation (4) is rearranged as eq. (15).   

\begin{equation} \ln x_{\text{Mn}} = -6\ln a_{\text{Al}} - \ln \gamma_{\text{Mn}}^{\text{o}} - \ln K_{(1)} \end{equation} (15)

The equilibrium constant K₍₁₎ at a given temperature is calculated by eqs. (2) and (3). The activity of Al and the activity coefficient of Mn at a given temperature and Mg content are calculated by eqs. (6) and eq. (14), respectively. The solubility of Mn in Al–Mg alloy at 823 and 873 K was calculated and shown in Fig. 5 with the experimental data. The calculated Mn solubility reasonably agrees with the experimental results, except that 19.8 and 35.5 mass%Mg at 873 K take smaller values than the calculated ones. The cause of this difference cannot be known, but it seems to be some error in the experiments.

Fig. 5

Solubility of manganese in molten Al–Mg alloy equilibrated with Al₆Mn at 823 and 873 K.

As mentioned in Section 3.2, the degree of freedom is 1 under constant temperature and pressure. Therefore, the equilibrium Mg content and temperature can be determined when the solubility of Mn is fixed. Equation (15) is solved numerically, and the temperature and Mg content of Al alloy at fixed Mn contents were derived. The results are described on the phase diagram of the Al–Mg binary system in Fig. 6. In Fig. 6, the bold line shows the liquidus of Al, and the thin lines are the derived contour lines of fixed Mn contents. The experimental results are also plotted in Fig. 6. The numbers in the figure indicate the mass percentage of Mn of each contour line and experimental plot. It is found that the contour lines reasonably agree with the experimental results except for the results of 19.8 and 35.5 mass%Mg, 0.47 and 0.11 mass%Mn, at 873 K. The removal limit of Mn can be estimated from the results. As can be seen, Mn content can be decreased at higher Mg content and lower temperature. This is caused by the increase in the equilibrium constant because the reaction is exothermic and the increase in the activity coefficient of Mn due to the repulsive interaction between Mn and Mg. Another benefit of Mg addition is the decrease in the liquidus temperature. Thermodynamic analysis showed the possibility of reducing the Mn content of Al to 0.0030 mass% at 733 K and 34 mass%Mg by the present removal method. The addition of Mg is proved to remove Mn effectively through precipitation of intermetallic compounds containing Mn.

Fig. 6

Contour lines of Mn content of molten Al–Mg alloy equilibrated with Al₆Mn.

4. Conclusion

Al–Mg molten alloy was equilibrated with Al₆Mn at 823 and 873 K, and the solubility of Mn was investigated. From the experimental results, thermodynamic data of the formation of Al₆Mn inter-metallic compounds were derived. Using the derived thermodynamic data, the reducing limit of Mn concentration was analyzed, and conclusions are summarized as follows:

1. (1)    Mn concentration of molten Al becomes lower at higher Mg content of molten Al and lower temperature. The Mn content was reduced to 0.07 mass% at 823 K and 38.2 mass%Mg.
2. (2)    The activity coefficient of Mn at each infinitely dilute solution of Al and Mg at 873 K was derived as follows:   
   \begin{equation*} \ln \gamma_{\text{Mn(${l}$,in Al)}}^{\text{o}} = -8.80,\quad \ln \gamma_{\text{Mn(${l}$,in Mg)}}^{\text{o}} = 8.67 \end{equation*}
3. (3)    The activity coefficient of Mn in molten Al–Mg alloy was increased at higher Mg content. It was derived as a function of temperature and molar fraction of Mg and is expressed as follows:   
   \begin{align*} &\ln \gamma_{\text{Mn(${l}$,in Al–Mg alloy)}}^{\text{o}} \\ &\quad = \frac{873}{T}(-0.459x_{\text{Mg}}{}^{2} + 17.94x_{\text{Mg}} - 8.80) \end{align*}
4. (4)    Mn content can be decreased at higher Mg content and lower temperature, which is caused by the increase in the equilibrium constant because the reaction is exothermic and the increase in the activity coefficient of Mn due to the repulsive interaction between Mn and Mg. Thermodynamic analysis showed that the Mn content of Al could be reduced to 0.0030 mass% at 733 K and 34 mass%Mg.

REFERENCES

• 1)  Japan Aluminum Association: Summary of LCI Data for New Aluminum Ingots and Recycled Ingots (2005).
• 2)   S.  Aoki: J. JILM 63 (2013) 260–270. doi:10.2464/jilm.63.260
• 3)  “Technology Road Map of Aluminum 2022” Japan Aluminium Association https://www.aluminum.or.jp/roadmap/pdf/2022.pdf (accessed 2022-05-20).
• 4)  T. Hiraki, L. Xin, K. Nakajima, K. Matsubae, S. Nakamura and T. Nagasaka: Proc. of the 23rd Annual Conf. of Japan Society of Material Cycles and Waste Management, (Japan Society of Material Cycles and Waste Management, 2012) pp. 269–270. https://doi.org/10.14912/jsmcwm.23.0_269.
• 5)   K.  Nakajima,  O.  Takeda,  T.  Miki,  K.  Matsubae,  S.  Nakamura and  T.  Nagasaka: Environ. Sci. Technol. 44 (2010) 5594–5600. doi:10.1021/es9038769
• 6)   T.  Hiraki,  T.  Miki,  K.  Nakajima,  K.  Matsubae,  S.  Nakamura and  T.  Nagasaka: Metals 7 (2014) 5543–5553. doi:10.3390/ma7085543
• 7)  “Patent Map of the Aluminum Recycling Technology” National Center for Industrial Property Information and Training, https://www.inpit.go.jp/blob/katsuyo/pdf/chart/fippan08.pdf (accessed 2022-05-20).
• 8)   M.  Okubo,  S.  Kumai and  K.  Ueda: J. MMIJ 123 (2007) 850–854. doi:10.2473/journalofmmij.123.850
• 9)  “Toward Government Strategies for Enhancing Material Innovation Power (Report Compiled by the Strategy Preparation Meeting)” Ministry of Economy, Trade and Industry, Japan https://www.meti.go.jp/press/2020/06/20200602002/20200602002-1.pdf (accessed 2022-05-20).
• 10)   C.  Chen,  J.  Wang,  D.  Shu,  P.  Li,  J.  Xue and  B.  Sun: Mater. Trans. 52 (2011) 1629–1633. doi:10.2320/matertrans.M2011108
• 11)  S. Mikubo: Proc. of the 12th Int. Conf. on Aluminium Alloys, (The Japan Inst. of Light Metals, 2010) pp. 224–228.
• 12)  M. Otaki, T. Soutome, K. Mori, H. Kudo and S. Tanaka: Furukawa Denkou Jihou, (104) (1999), 25–30. https://www.furukawa.co.jp/jiho/fj104/fj104_07.pdf.
• 13)   H.L.  Moraes,  J.R.  Oliveira,  D.C.R.  Espinosa and  J.A.S.  Tenório: Mater. Trans. 47 (2006) 1731–1736. doi:10.2320/matertrans.47.1731
• 14)   M.  Gnatko,  C.  Li,  A.  Arnold and  B.  Friedrich: Metals 8 (2018) 796–808. doi:10.3390/met8100796
• 15)  Y. Shinomiya, J. Yamamoto, K. Kato, H. Ono, K. Yamaguchi and K. Komori: Collected Abstracts of the 2022 Spring Meeting of the Japan Inst. Light Metals, (2022) pp. 207–208.
• 16)  Y. Sekii, J. Yamamoto, K. Kato, H. Ono, K. Yamaguchi and K. Komori: Collected Abstracts of the 2022 Spring Meeting of the Japan Inst. Light Metals, (2022) pp. 211–212.
• 17)   A.J.  McAlister and  J.L.  Murray: J. Phase Equilibria 8 (1987) 438–447. doi:10.1007/BF02893153
• 18)   X.J.  Liu,  I.  Ohnuma,  R.  Kainuma and  K.  Ishida: J. Phase Equilibria 20 (1999) 45–56. doi:10.1361/105497199770335938
• 19)  B. Predel: Phase Equilibria, Crystallographic and Thermodynamic Data of Binary Alloys, Landolt-Börnstein, Numerical Data and Functional Relationships in Science and Technology, New Series/Editors in Chief: O. Madelung and W. Martienssen, Group IV: Macroscopic Properties of Matter, Volume 5, (Springer-Verlag, Berlin, 1991) pp. 160–163.
• 20)   M.  Zheng and  Z.  Kozuka: J. Japan Inst. Metals 51 (1987) 44–50. doi:10.2320/jinstmet1952.51.1_44
• 21)   J.  Moriyama,  Z.  Kozuka and  T.  Oishi: Bull. Japan Inst. Metals 9 (1970) 764–776. doi:10.2320/materia1962.9.764
• 22)   H.  Ono-Nakazato,  D.  Hirai and  T.  Usui: J. High Temp. Soc. 26 (2000) 139–144.
• 23)  H.L. Lukas: COST 507 Thermochemical Database for Light Metal Alloys, ed. by I. Ansara, A.T. Dinsdale and M.H. Rand, (EUR 18499 EN 2, 1998) 215–217.
• 24)   N.  Satoh,  T.  Taniguchi,  S.  Mishima,  T.  Oka,  T.  Miki and  M.  Hino: Tetsu-to-Hagané 95 (2009) 827–836. doi:10.2355/tetsutohagane.95.827