
- 3 号 p. 409-
- 2 号 p. 203-
- 1 号 p. 1-
- |<
- <
- 1
- >
- >|
-
Makiko Mase2019 年 42 巻 3 号 p. 409-430
発行日: 2019/10/31
公開日: 2019/11/06
ジャーナル フリーWe study families of K3 surfaces obtained by double covering of the projective plane branching along curves of (2,3)-torus type. In the first part, we study the Picard lattices of the families, and a lattice duality of them. In the second part, we describe a deformation of singularities of Gorenstein K3 surfaces in these families.
抄録全体を表示PDF形式でダウンロード (349K) -
Takashi Suzuki2019 年 42 巻 3 号 p. 431-475
発行日: 2019/10/31
公開日: 2019/11/06
ジャーナル フリーIn this paper, we propose a definition of Néron models of arbitrary Deligne 1-motives over Dedekind schemes, extending Néron models of semi-abelian varieties. The key property of our Néron models is that they satisfy a generalization of Grothendieck's duality conjecture in SGA 7 when the residue fields of the base scheme at closed points are perfect. The assumption on the residue fields is unnecessary for the class of 1-motives with semistable reduction everywhere. In general, this duality holds after inverting the residual characteristics. The definition of Néron models involves careful treatment of ramification of lattice parts and its interaction with semi-abelian parts. This work is a complement to Grothendieck's philosophy on Néron models of motives of arbitrary weights.
抄録全体を表示PDF形式でダウンロード (325K) -
Ryota Hirakawa2019 年 42 巻 3 号 p. 476-495
発行日: 2019/10/31
公開日: 2019/11/06
ジャーナル フリーThis is the second of our series of papers to solve Mutsuo Oka's problems concerning our polyhedral construction of degenerations of Riemann surfaces. Oka posed globalization problem of our degenerations and determination problem of the defining equation of a Riemann surface appearing in our construction—which is equipped with the standard tetrahedral group action (i.e. topologically equivalent to the tetrahedral group action on the cable surface of the tetrahedron). A joint work with S. Takamura solved the first problem. In this paper, we solve the second one—in an unexpected way: an algebraic curve with the standard tetrahedral group action turns out to be not unique: a sporadic one (hyperelliptic) and a 1-parameter family of non-hyperelliptic curves. We study their properties. At first glance they are `independent', but actually intricately connected—we show that at one special value in this family, a degeneration whose monodromy is a hyperelliptic involution occurs, and the sporadic hyperelliptic curve emerges after the stable reduction (hyperelliptic jump). This jumping phenomenon seems deeply related to the moduli geometry and is possibly universal for other families of curves with finite group actions. Based on this observation, we pose stably-connectedness problem.
抄録全体を表示PDF形式でダウンロード (377K) -
Atsufumi Honda, Kentaro Saji2019 年 42 巻 3 号 p. 496-525
発行日: 2019/10/31
公開日: 2019/11/06
ジャーナル フリーWe introduce two invariants called the secondary cuspidal curvature and the bias on 5/2-cuspidal edges, and investigate their basic properties. While the secondary cuspidal curvature is an analog of the cuspidal curvature of (ordinary) cuspidal edges, there are no invariants corresponding to the bias. We prove that the product (called the secondary product curvature) of the secondary cuspidal curvature and the limiting normal curvature is an intrinsic invariant. Using this intrinsicity, we show that any real analytic 5/2-cuspidal edges with non-vanishing limiting normal curvature admit non-trivial isometric deformations, which provides the extrinsicity of various invariants.
抄録全体を表示PDF形式でダウンロード (414K) -
Yuichi Shimada2019 年 42 巻 3 号 p. 526-565
発行日: 2019/10/31
公開日: 2019/11/06
ジャーナル フリーLet F be a totally real field and ρ = (ρλ)λ be a compatible system of two dimensional λ-adic representations of the Galois group of F. We assume that ρ has a residually modular λ-adic realization for some λ. In this paper, we consider local behaviors of modular deformations of λ-adic realizations of ρ at unramified primes. In order to control local deformations at specified unramified primes, we construct certain Hecke modules. Applying Kisin's Taylor-Wiles system, we obtain an R = T type result supplemented with local conditions at specified unramified primes. As a consequence, we shall show a potential rigidity of some modular deformations of infinitely many λ-adic realizations of ρ.
抄録全体を表示PDF形式でダウンロード (362K) -
Junjiro Noguchi2019 年 42 巻 3 号 p. 566-586
発行日: 2019/10/31
公開日: 2019/11/06
ジャーナル フリーThe proofs of K. Oka's Coherence Theorems are based on Weierstrass' Preparation (division) Theorem. Here we formulate and prove a Weak Coherence Theorem without using Weierstrass' Preparation Theorem, but only with power series expansions: The proof is almost of linear algebra. Nevertheless, this simple Weak Coherence Theorem suffices to give other proofs of the Approximation, Cousin I/II, and Levi's (Hartogs' Inverse) Problems even in simpler ways than those known, as far as the domains are non-singular; they constitute the main basic part of the theory of several complex variables.
The new approach enables us to complete the proofs of those problems in quite an elementary way without Weierstrass' Preparation Theorem or the cohomology theory of Cartan-Serre, nor L2-
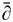 method of Hörmander.
method of Hörmander.We will also recall some new historical facts that Levi's (Hartogs' Inverse) Problem of general dimension n ≥ 2 was, in fact, solved by K. Oka in 1943 (unpublished) and by S. Hitotsumatsu in 1949 (published in Japanese), whereas it has been usually recognized as proved by K. Oka 1953, by H. J. Bremermann and by F. Norguet 1954, independently.
抄録全体を表示PDF形式でダウンロード (209K) -
Makoto Abe, Gou Nakamura, Hiroshige Shiga2019 年 42 巻 3 号 p. 587-592
発行日: 2019/10/31
公開日: 2019/11/06
ジャーナル フリーIn this paper, we give a topological characterization of a subdomain G of an open Riemann surface R which has the strong disk property. Namely, we show that the domain G satisfies the strong disk property in R if and only if the canonical homomorphism π1(G) → π1(R) is injective.
抄録全体を表示PDF形式でダウンロード (76K) -
Kazumasa Inaba2019 年 42 巻 3 号 p. 593-610
発行日: 2019/10/31
公開日: 2019/11/06
ジャーナル フリーLet f be a 1-variable complex polynomial such that f has an isolated singularity at the origin. In the present paper, we show that there exists a perturbation ft of f which has only fold singularities and cusps as singularities of a real polynomial map from R2 to R2. We then calculate the number of cusps of ft in a sufficiently small neighborhood of the origin and estimate the number of cusps of ft in R2.
抄録全体を表示PDF形式でダウンロード (158K) -
Ohsang Kwon, Youngae Lee2019 年 42 巻 3 号 p. 611-632
発行日: 2019/10/31
公開日: 2019/11/06
ジャーナル フリーIn this paper, we consider a nonautonomous quasilinear equation with general nonlinearity. Our main goal is to show the existence and asymptotic behavior of solutions with small parameter, which correponds to the bifurcation from infinity.
抄録全体を表示PDF形式でダウンロード (179K)
- |<
- <
- 1
- >
- >|